10th
European Intensive Course on Complex Analysis
and
applications to partial differential
equations
|
|
Departamento de Matemática,
Universidade de Coimbra, Portugal
May 31 to June 11 2004
|
|
|
| Goal of the Course |
|
This intensive course follows the nine held
at the Universities of Coimbra
and Aveiro from 1995 to 2003 and there are plans for intensive courses
in the following years. The lecture
notes of some of the courses have been published in Coimbra and
others are in print.
This
intensive course will have a total of 40 hours of lectures and is at
postgraduate level. Lecturers will have time available to discuss with
the students. Successfully participating students will get a
certificate. This course is organized by the Universities of Coimbra and
Aveiro with the same goals as the ones organized under the Socrates/Erasmus
Intensive Program of Higher Education, and is opened to all young mathematicians interested in Complex
Analysis and its applications.
There
will be a Workshop
on "Applications and Generalizations of Complex Analysis" on
the 4th and 5th of June 2004.
In
the occasion of the 10th European Intensive Course on Complex
Analysis, this Workshop will be in honor of the coordinators of the
Erasmus Galois Network that sponsored this series of Intensive Courses, and the
following distinguished
Professors
for theirs contributions in this series of Intensive Courses, Richard Delanghe (Ghent University, Belgium), Sean
Dineen (University College Dublin, Ireland), José Fernandes de
Carvalho (Coimbra University, Portugal), Jean de Graaf
(University of Eindhoven, Netherlands), Gerhard
Jank (RWTH Aachen, Germany), and Francisco Marcellán (Carlos
III University, Spain). |
|
|
|
First Week
|
| |
May
31 |
June
01 |
June
02 |
June
03 |
| Opening
session (Room 2.3) |
9h30m-10h |
|
|
|
| Van
Assche (Room 2.3) |
10h-12h30m |
10h-12h30m |
10h-12h30m |
10h-12h30m |
| Martínez Finkelshtein
(Room 2.4) |
14h30m-17h |
14h30m-17h |
14h30m-17h |
14h30m-17h |
|
de Graaf
(Room 2.4) |
|
|
17h-18h |
17h-18h |
|
|
Second Week
|
| |
June
07 |
June 08 |
June 09 |
June 11 |
| Mackey
(Room 2.3) |
10h-12h30m |
10h-12h30m |
10h-12h30m |
10h-12h30m |
| Bernstein
(Room 2.3) |
14h30m-17h |
14h30m-17h |
14h30m-17h |
14h30m-17h |
|
de Graaf
(Room 2.3) |
17h-18h |
17h-18h |
|
|
|
|
|
|
|
| Title: Potential
Theory |
| Summary: The
course will cover the main properties of the logarithmic potential up to
the proof of the existence of the equilibrium measure. Applications. |
|
|
| Title:
Wavelet Transforms as Frame Transforms |
| Summary: |
|
|
|
| Title: The Schwarz lemma and Composition Operators |
| Summary: Starting with the famous Schwarz lemma we examine metric properties of
holomorphic functions on the unit disc, D, and look at the connection
between the Poincaré metric on D and the operator norm for composition
operators on the space of bounded holomorphic functions on D. |
|
|
| Title:
Borel-Pompeiu's Formulae in Complex and Clifford Analysis |
Summary: Function
theory is the theory of functions of one complex variable
and the central operator is the so-called
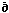 -operator which describes the Cauchy-Riemann
differential operator. -operator which describes the Cauchy-Riemann
differential operator. |
| An
important tool to study the analytic properties of holomorphic functions
is the Borel-Pompeiu formula, which is in fact a representation
formula. From the Borel-Pompeiu formula the Cauchy formula for
holomorphic functions can be derived as well as the formulation of the
Riemann-Hilbert problem in terms of a singular integral equation. But
the same theory can be developed using a perturbed Cauchy-Riemann
differential operator. We will evolve Borel-Pompeiu's formulae for the
perturbed Cauchy-Riemann differential operator and deduce
Plemelj-Sokhotzki's formulae and the Hardy spaces. In order to obtain
similar formulae in the space or even higher dimensions we introduce the
algebra of quaternions and Clifford algebras. We will show
that the Dirac operator gives a higher dimensional analogue to
the Cauchy-Riemann operator. We will prove Borel-Pompeiu's
formulae again for this operator. |
|
|
| Title: Riemann-Hilbert problem for orthogonal polynomials |
Summary: A Riemann-Hilbert problem consists of finding an
analytic function in the complex plane minus a collection of oriented contours, for which the boundary values on the contours
(from both sides of the contours) are given. Typically the solution will involve the Cauchy transform of a function
w on the contours, where w describes the boundary values on the contours. The basic idea of the Riemann-Hilbert
approach to orthogonal polynomials is to characterize orthogonal polynomials corresponding to a weight function
w on the real line via a boundary value problem for matrix valued
analytic functions. This Riemann-Hilbert problem was first formulated by Fokas, Its and Kitaev in 1992 and we will formulate
the appropriate Riemann-Hilbert problem for orthogonal polynomials on ]-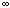 ,+ ,+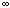 [,
[0,+ [,
[0,+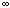 [ and [-1,1]. We show that this Riemann-Hilbert problem enables us to find the
three-term recurrence relation and the differential equation for Hermite polynomials, Laguerre polynomials, and Jacobi
polynomials. One of the main advantages of this Riemann-Hilbert approach is that it allows to obtain strong uniform asymptotics
which is valid in the whole complex plane. The idea is to transform the initial Riemann-Hilbert problem in a few steps to another
equivalent Riemann-Hilbert problem on a deformed set of contours with simpler jumps on the contours. This technique is called
the steepest descent method for Riemann-Hilbert problems and was developed by Deift and Zhou in 1993. If time permits we will
show how it works to obtain the strong asymptotics for Laguerre polynomials. [ and [-1,1]. We show that this Riemann-Hilbert problem enables us to find the
three-term recurrence relation and the differential equation for Hermite polynomials, Laguerre polynomials, and Jacobi
polynomials. One of the main advantages of this Riemann-Hilbert approach is that it allows to obtain strong uniform asymptotics
which is valid in the whole complex plane. The idea is to transform the initial Riemann-Hilbert problem in a few steps to another
equivalent Riemann-Hilbert problem on a deformed set of contours with simpler jumps on the contours. This technique is called
the steepest descent method for Riemann-Hilbert problems and was developed by Deift and Zhou in 1993. If time permits we will
show how it works to obtain the strong asymptotics for Laguerre polynomials. |
|
|
|
Living expenses can be partially covered for some
students if they do not have support from their own institution and if
there is enough money available.
|
|
|
|
|
|
|
|
Abreu, Luis Daniel Moura. Address.
Departamento de Matemática, FCTUC, Apartado 3008, 3000 Coimbra, Portugal. e.mail.
daniel@mat.uc.pt. |
|
Almeida, Regina. Address.
UTAD, Dep. de Matemática, Quinta de Prados, Apartado 1013, 5000-911
VILA REAL. e.mail.
ralmeida@utad.pt. |
| Bernstein,
Sanhild. address. Bauhaus-Universität Weimar, Mathematische
Optimierung, Coudraystr. 13B, 99421 Weimar,
Germany. e.mail. swanhild.bernstein@fossi.uni-weimar.de. |
| Carvalhal,
Gonçalo Nuno de Almeida de Sousa Teles do. address. Ladeira das Alpenduradas, nº42 3030 Coimbra.
e.mail. GCarvalhal@netcabo.pt. |
| Cotrim,
Luís. address. Escola Superior de Tecnologia e Gestão do
I.P.L., Quinta do Seixo lote 1 - 4 Dto 2410 Leiria, Portugal. e.mail.
lmsc@estg.ipleiria.pt. |
|
Coutinho, Cláudia Alexandra. address.
Departamento de Matemática da Universidade de Aveiro, Campus de Santiago,
3810 Aveiro.
e.mail. ccoutinho@prof2000.pt. |
|
Ferreira, Milton dos Santos. address.
Departamento de Matemática da Universidade de Aveiro, Campus de Santiago,
3810 Aveiro.
e.mail. mferreira@mat.ua.pt. |
| de
Graaf, Johannes. address. Santvlietmolen 3, 5612 MD EINDHOVEN, Nederland.
e.mail. J.d.Graaf@tue.nl. |
| Horan,
David. address. Dept. of Mathematics, UCD, Belfield, Dublin 4,
Ireland. e.mail. |
| Jain,
Rahul. address. Tata Institute of Fundamental Research(TIFR), Indian Institute of Science
(IISc), Bangalore, India. e.mail. rahul@math.tifrbng.res.in |
| Jesus,
Márcio Dinis do Nascimento de. address. Instituto Politecnico de Viseu,
Escola Superior e Tecnologia de Viseu. e.mail. mnasce@mat.estv.ipv.pt. |
|
Loureiro Vieira, Nelson Felipe. address.
Departamento de Matemática da Universidade de Aveiro, Campus de Santiago,
3810 Aveiro.
e.mail. nvieira@mat.ua.pt. |
| Martinez-Finkelshtein,
Andrei. address. Dept. Estadistica y Matematica Aplicada, Universidad de Almeria, La Canada, 04120
Almeria, Spain. e-mail: andrei@ual.es. |
| Mendes,
Ana Isabel. address. Escola Superior de Tecnologia e Gestão
do I.P.L., Quinta do Seixo lote 1 - 4 Dto 2410 Leiria, Portugal. e.mail.
aimendes@estg.ipleiria.pt. |
| Mackey,
Michael. address. Dept. of Mathematics, UCD, Belfield, Dublin 4,
Ireland. e.mail. Michael.Mackey@ucd.ie. |
| Monteiro,
Paulo Alexandre Bessa Soares. address. Tv. dos Xistos nº 22, 4435-826 BAGUIM DO MONTE.
e.mail. anthrax@clix.pt. |
| Nogueira,
João Manuel. address. Departamento de Matemática, FCTUC,
Apartado 3008, 3000 Coimbra, Portugal. e.mail. nogueira@mat.uc.pt. |
| O'Shea,
James. address. Dept. of Mathematics, UCD, Belfield, Dublin 4,
Ireland. e.mail. |
| Paiva,
Anabela Monteiro. address. Universidade da Beira Interior,
Departamento de Matemática, Av. Marquês D'Ávila e Bolama, 1, Covilhã.
e.mail. apaiva@noe.ubi.pt. |
| Pereira
de Sousa, Victor Luís. address. Instituto Politécnico
de Bragança, Escola Superior de Tecnologia e de Gestão de Bragança,
Ap. 134, 5301-857 Bragança, Portugal. e.mail. vitorsousa@ipb.pt. |
| Rebocho,
Maria das Neves. address. Universidade da Beira Interior,
Departamento de Matemática, Av. Marquês D'Ávila e Bolama, 1, Covilhã.
e.mail. mneves@noe.ubi.pt. |
| Santos,
Ana Margarida. address. Universidade de Aveiro, Departamento
de Matemática, Campus de Santiago, 3810 Aveiro, Portugal. e.mail.
asantos@mat.ua.pt. |
|
Santos Cardoso, José Luis.
address. UTAD, Dep. de Matemática, Quinta de Prados, Apartado 1013,
5000-911 VILA REAL. e.mail. jluis@utad.pt. |
| Vicente Majúa,
Julio Iñigo de. address.Universidad Carlos III, Ave.
Universidad, 30, 28911 Leganés-Madrid, Spain. e.mail. |
| Van
Assche, Walter. address.
Katholieke Universiteit Leuven, Celestijnenlaan 200 B 3001 Leuven,
Belgium. e.mail.walter@wis.kuleuven.ac.be. |
|
|
|
|
|
|
| With
support from CMUC
(Centro
de Matemática da Universidade de Coimbra), UI&D "Matemática e
Aplicações" da Universidade de Aveiro, and the Socrates programme |

|
 |
 |


